These triangles are special triangles because the ratio of their sides are known to us so we can make use of this information to help us in right triangle trigonometry problems In the case of the triangle, their side's ratios are 1 2 3 \sqrt3 3Right Triangle Calculator Although all right triangles have special features – trigonometric functions and the Pythagorean theorem The most frequently studied right triangles , the special right triangles, are the 30, 60, 90 Triangles followed by the 45, 45, 90 triangles2 n = 2 × 4 = 8 Answer The length of the hypotenuse is 8 inches You can also recognize a triangle by the angles As long as you know that one of the angles in the rightangle triangle is either 30° or 60° then it must be a special right triangle

30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com
Right triangle 30 60 90 calculator
Right triangle 30 60 90 calculator- A triangle is a special type of right triangle that has a 30degree angle and a 60degree angle in addition to the right angle This triangle hasUse this value to label the other two sides of the triangle Use the values on the triangle to




30 60 90 Triangle Explanation Examples
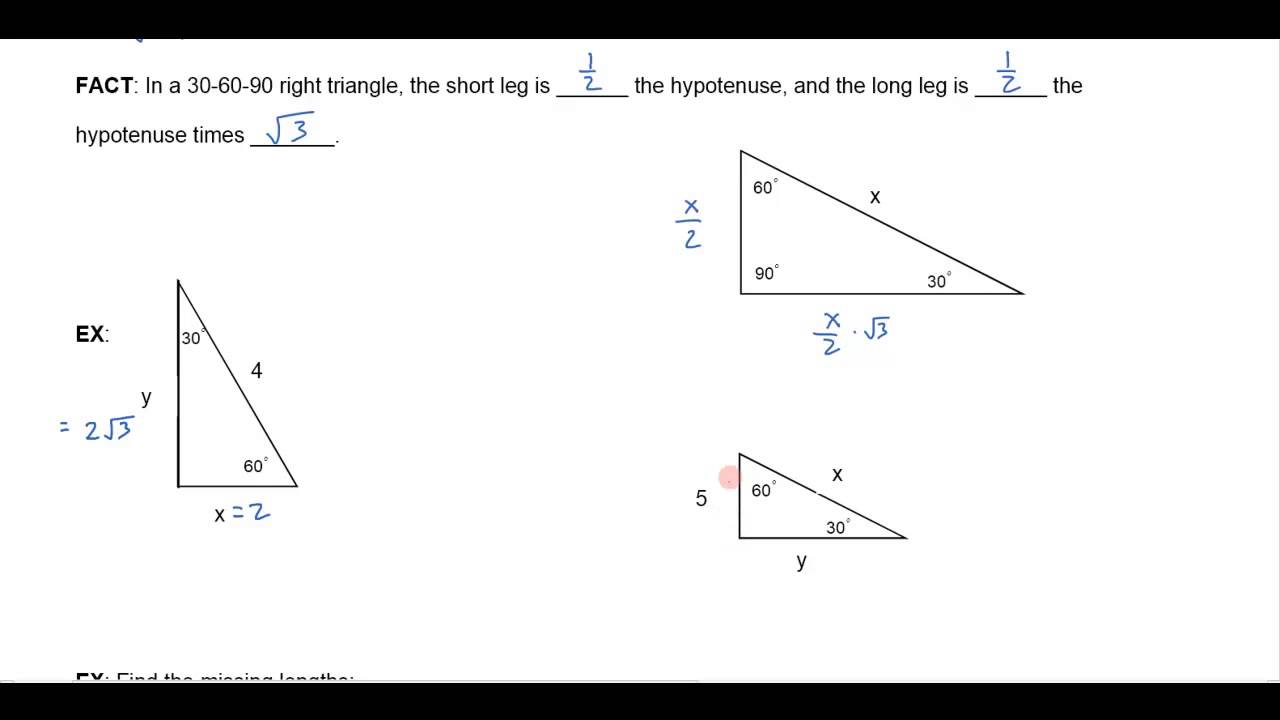
THE 30°60°90° TRIANGLE THERE ARE TWO special triangles in trigonometry One is the 30°60°90° triangle The other is the isosceles right triangle They are special because, with simple geometry, we can know the ratios of their sides Theorem In a 30°60°90° triangle the sides are in the ratio 1 2 We will prove that below• area = 05 * long side * short side;A 30 ° − 60 ° − 90 ° triangle is commonly encountered right triangle whose sides are in the proportion 1 3 2 The measures of the sides are x , x 3 , and 2 x In a 30 ° − 60 ° − 90 ° triangle, the length of the hypotenuse is twice the length of the shorter leg, and the length of the longer leg is 3 times the length of the shorter leg
A triangle is a special right triangle with some very special characteristics If you have a degree triangle, you can find a missing side length without using the Pythagorean theorem!Check out this tutorial to learn about triangles! A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one another
Represents the angle measurements of a right triangle This type of triangle is a scalene right triangle The sides are in the ratio of , with the across from the 30, the as the hypotenuse, and the across from 60 Using variables, it can be written as The two special right triangles are right triangles with interior angles measuring 30 60 90 and 45 45 90 What is the 45 45 90 triangle rule?A triangle where the angles are 30°, 60°, and 90° Try thisIn the figure below, drag the orange dots on each vertexto reshape the triangle Note how the angles remain the same, and it maintains the same proportions between its sides This is one of the 'standard' right trianglesyou should be able recognize on sight



Trigonometry




The Complete Guide To The 30 60 90 Triangle
• long side = hypotenuse * sin(60̊);What I want to do in this video is discuss a special class of triangles called triangles and I think you know why they're called this the measures of its angles are 30 degrees 60 degrees and 90 degrees and what we're going to prove in this video this tends to be a very useful result at least for a lot of what you see in a geometry class and then later on in trigonometry class is theLearn how to solve for the sides in a Special Right Triangle in this free math video tutorial by Mario's Math Tutoring009 What are the Ratios of t




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com



The Easy Guide To The 30 60 90 Triangle
A right triangle is a special type of right triangle 30 60 90 triangle's three angles measure 30 degrees, 60 degrees, and 90 degrees The triangle is significant because the sides exist in an easytoremember ratio 1√ (3/2) This means that the hypotenuse is twice as long as the shorter leg and the longer leg is the square root ofThe 45 45A triangle is a special right triangle with some very special characteristics If you have a degree triangle, you can find a missing side length without using the Pythagorean theorem!



Solving A 30 60 90 Right Triangle Youtube




30 60 90 Right Triangles Solutions Examples Videos
Watch more videos on http//wwwbrightstormcom/math/geometrySUBSCRIBE FOR All OUR VIDEOS!https//wwwyoutubecom/subscription_center?add_user=brightstorm2VITriangles are classified as "special right triangles" They are special because of special relationships among the triangle legs that allow one to easily arrive at the length of the sides with exact answers instead of decimal approximations when using trig functionsIt is a triangle where the angles are always 30, 60 and 90 As one angle is 90, so this triangle is always a right triangle As explained above that it is a special triangle so it has special values of lengths and angles The basic triangle sides ratio is The side opposite the 30° angle x



Right Triangle 30 60 90 Problems And Answer Key Tpt




Special Right Triangles Review Article Khan Academy
100 The special right triangle that is formed by drawing the diagonal of a square What is a triangle? Get Your Chances The triangle is a special right triangle, and knowing it can save you a lot of time on standardized tests like the SAT and ACT Because its angles and side ratios are consistent, test makers love to incorporate this triangle into problems, especially on the nocalculator portion of the SAT A triangle is a unique right triangle that contains interior angles of 30, 60, and also 90 degrees When we identify a triangular to be a 30 60 90 triangular, the values of all angles and also sides can be swiftly determined Imagine reducing an equilateral triangle vertically, right down the middle




30 60 90 Triangle Calculator Formula Rules




30 60 90 Triangles



0 件のコメント:
コメントを投稿